Math 450 – An Introduction to Real
Analysis – 3 credits
Professor: M.
Greeley
Office:
Telephone: 617 287 6456
Office Hours:
Tu/Thu 11-12 or by appointment
Course description: A rigorous treatment of the calculus of functions of one real variable. Emphasis is on proofs. Includes discussion of topology of real line, limits, continuity, differentiation, integration and series.
Prerequisite: Math 310 or Math 354
Textbook: Analysis with an Introduction to Proof, by Steven R. Lay
Homework: Due the following week on Thursday. Homework must look “professional”; i.e. no scratch-work, use rulers or graphing paper where appropriate.
Grade: Homework 20%, Midterm 40%, Final Exam 40%
Section Topic
10 Natural Numbers and Induction
11 Ordered Fields
12 The Completeness Axiom
13 Topology of the Reals
14 Compact Sets
16 Convergence
17 Limit Theorems
18 Monotone Sequences and Cauchy Sequences
19 Subsequences
20 Limits of Functions
21 Continuous Functions
22 Properties of Continuous Functions
23 Uniform Continuity
25 The Derivative
26 The Mean Value Theorem
27 L’Hopital’s Rule
28
29 The Riemann Integral
30 Properties of the Riemann Integral
31 The Fundamental Theorem of Calculus
32 Convergence of Infinite Series
33 Convergence Tests
34 Power Series
35 Pointwise and Uniform Convergence
36 Applications of Uniform Convergence
37 Uniform Convergence of Power Series
Homework Assignments:
Due Page
2/4 91: 4*, 8*, (13c)*, 16*
2/11 102: 1,2,(3cj)*, 4*, 10*,( Why can’t Theorem 12.7 be applied be applied to 12.2a?)*, (12.2ab)*
2/18 112: 1, 2*, 6*, 10*, 12*
2/26 120: 1, 3, 4*, 5, 6*, 8*, 16*
![]()
127:
2*, 3, 4*, 8*, 12* (Hint on 12: 1st, show it’s closed by showing
that ![]() and 2d show that it’s
bounded (if not, then the infinite
and 2d show that it’s
bounded (if not, then the infinite
subset property will be violated)
3/4 146: 1, 2*, 4*, 6*, 10*
(1)* ![]() (use the definition of
convergence)
(use the definition of
convergence)
(2) 10.14* (Hint: use induction)
3/11 154: 2*, 6*, 9*, 16*
3/25 161: 1, (2ab)*, 4*, 6*, 10*
168: 1, 2*, 8*
4/1 177: 2*, 4*, 5c, 6*, 9* (in your own words!)
4/8 186: 2*, 6*, 10*, 11* (in your own words), 16*
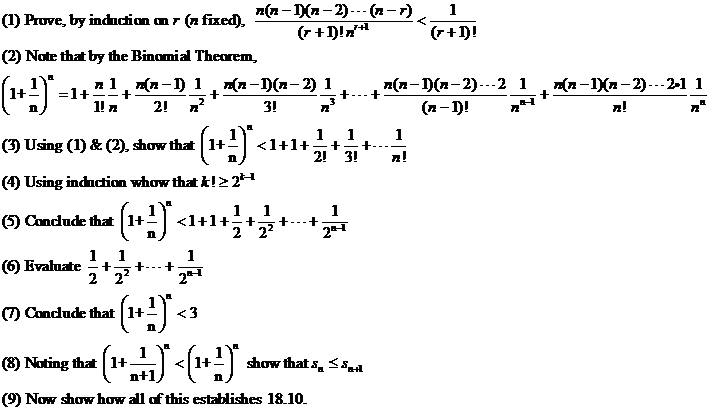
4/15 (16.13)*
192: 2*, 4*, 6*, 11*, 12*
199: 2*, (4ab)*, 6*
4/22 216: 2*, 6*, 10*
224: 2*, 4*, (5acfh)*, 25.6*, 12*
4/29 249: 2*, 4*, 6*, 11*, 14*
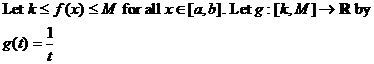
also 11*
264: 4*, 6*, 10*, 16*